Associative Thickener

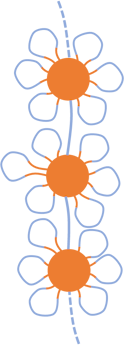
Here is a HEUR (Hydrophobically Modified Ethoxylated Urethane) molecule, highly stylised. The hydrophobic (alkyl) chains [orange] are attached to either end of an ethoxylate chain [blue]. This "telechelic" form is created by linking up [green blobs] the two end alcohols of the ethoxylate with two long-chain alcohols via an isocyanate reaction, creating a urethane. They can accumulate to form "flower micelles" with each micellar core simplified as an orange blob. These flower micelles can join up (one end from one micelle the other in the next micelle) into long superbridged structures which create the entangled network giving the desired high viscosity of these thickeners.

However, with a bit of shear, the network falls apart, leading to normal low viscosities. When the shear is removed, the network can rapidly re-assemble, returning to a high viscosity and yield strength.

The graph shows this concentration dependence. We see that at a critical entanglement concentration C* the low-shear viscosity, which increased linearly with concentration of surfactant, starts to increase strongly, to the power 3.5, so you get a lot of viscosity for a little extra HEUR. Just like a real polymer, there's a relaxation time, τ_rep based on reptation physics which causes this strong dependency. However, because the micelles easily break and reform there is another timescale τ_b. Whether you see easily-broken chains or polymer-like behaviour depends on the actual relaxation time, τ_R which is often considered to be given by `τ_R=sqrt(t_b . t_(rep)`. This really shows up in the oscillatory rheology via G' and G'' which are described, at frequency ω, by a pure Maxwell equation based around a base value, G₀:

`G'=G_0(ω^2τ_R^2)/(1+ω^2τ_R^2)`

`G''=G_0(ωτ_R)/(1+ω^2τ_R^2)`

The value of G₀ is in turn concentration dependent, being linear below C* then to the 2.25 power above it. τ_R has only a 1.25 power dependency. The app captures all these factors. You can choose to see the values in linear or log form. My personal preference is for linear, but the standard form is log.

The length of the ethoxylate chain, the lengths of the alkane chains, and the chemistry used to create the links each have complicated effects on the properties. For example, going from C14 to C18 might increase `τ_R` 100x and you would expect the viscosity to also increase, which it does for a given molar concentration. But we tend to use wt% in formulations so large MWt decreases the number of molecular units so there are some trade-offs. Changing the linking chemistry effects the dynamics, the hydrophobic balance, the MWt and also the polydispersity of the system - the more polydisperse the less precise the control, as discussed below with the HASEs.

The zero shear viscosity, η, is derived directly from the core values in the Maxwell equation, `η=G_0τ_R`. This calculated value is shown.

The point is that the behaviour of these micelles is extremely timescale-dependent. Over long timescales, they are low modulus, low viscosity. Over short timescales they can behave like super-high molecular weight polymers because their chains can be μm long! Of course, this behaviour is destroyed by shear, otherwise they would be unusable.

Flows like honey, wobbles like jelly

As explained by Neil Cunningham of the Centre for Industrial Rheology, the implications of the right-hand graph are that at low shear rates, gently tipping a bottle, the associative thickener formulation should flow like honey (or, as in his excellent TA Webinar , corn syrup), while at high speeds it is elastic and wobbles like jelly.

HASEs

Equally as popular as the HEURs are the HASEs, Hydrophobic Alkali-Swellable Emulsion Polymers. They show the same behaviour but their equivalent form of association is more polydisperse than the HEURs. So the ω in the Maxwell equation is replaced by `ω^α` for G' and `ω^β` for G''. With α and β decreasing from the HEUR's value of 1 down to as low as 0.5, you get a less sharp set of curves. If a sharper frequency and concentration response are of great concern to you then HEURs may be better, otherwise you decide based on other factors.

Surfactant effects

If you add extra surfactant to the system the G' and low shear viscosity may well increase, though it soon reaches a maximum then decreases. The reason seems to be that the surfactant can kick out a tail from one of the flower micelles, freeing it up to create new links and a stronger network. Eventually extra surfactant starts to compete even with linkages, so things get worse. The real life situation is complicated by the fact that your formulation, before adding the HEUR, may already contain some free surfactant - so the same HEUR at the same concentration might give different thickening effects for two similar formulations where one (perhaps unknown to you) has a higher free concentration of surfactant. HEURs are often full of surprises.

As kindly pointed out by Dr Joachim Venzmer, although adding general surfactants leads to modest effects, smarter choices of "pre-wormlike" surfactants, with Critical Packing Parameters ~ 1 can produce more powerful synergistic effects. A description of the technique from Venzmer and colleagues can be found in Thickening Agents for Surfactant Systems.

Using the app

You enter relaxation time τ_R and G₀ at C*, along with C* itself and C_max for the X axis. In the left-hand graph you then see how the viscosity (calculated from τ_R and G₀) changes and how G₀ changes with concentration. In the right-hand graph you see the expected rheology at your chosen C value (the C for G slider) for the rheology plot. This is a reminder that the properties of associative thickeners depend very strongly on the speed at which you measure them.

What values to use for the C*, τ_R, E_aand G₀ values? There is no way to know these in advance. Instead, use the app to find these values by getting a good-enough fit to whatever experimental data you have.

Acknowledgement

I was lost in HEUR science till I found the wonderful review by Ronald G. Larson, Antony K. Van Dyk Tirtha Chatterjee, Valeriy V. Ginzburg, Associative thickeners for waterborne paints: Structure, characterization, rheology, and modeling, Progress in Polymer Science 129 (2022) 101546. I've necessarily simplified a complex story, so blame me for errors. Reviews of this quality are all too rare and I pass my warm thanks to the authors.